Due to an omission in recent editions of The Intelligent Investor, analysts often recommend stocks today with a formula that Graham intended as a warning.
Introduction
Benjamin Graham was Warren Buffett's professor and mentor at Columbia Business School. Buffett even named his son - Howard Graham Buffett - after Graham. In the preface to Graham's book - "The Intelligent Investor" - Buffett calls it "by far the best book about investing ever written."
GrahamValue applies Graham's 17 financial criteria to 4500 NYSE and NASDAQ stocks to find Defensive, Enterprising and NCAV grade Graham stocks today.
Today, we will look into a formula that Graham actually warned against but is widely recommended as the "Benjamin Graham Formula", how this confusion came about, and what Graham actually wrote.
The Wrong Intrinsic Value Formula
The formula itself is mentioned in "Chapter 11: Security Analysis for the Lay Investor" of Graham's seminal book "The Intelligent Investor" as:
Value = Current (Normal) Earnings X (8.5 plus twice the expected annual growth rate)

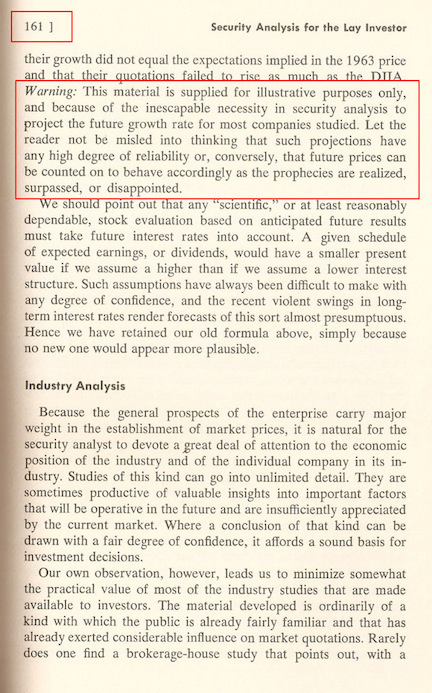
As shown in the scan above, Graham intended this formula to produce figures close to formal appraisals related to the valuation of growth stocks.
He then uses this formula to make the converse calculation of determining what rates of growth are anticipated by the current market price of a given stock, and then explains why such expectations are almost always unrealistic.
The Cause of the Confusion
What seems to have started the confusion is that the most commonly available edition of the book today is not the one originally written by Graham, but the new version with commentary by Jason Zweig.

In this edition, all the Foot Notes from the original book have been moved to the end of the book to make place for Zweig's commentary. For example, if we look at the same page with the formula in the original book, we see the Foot Note where Graham cautions against using this formula.

But in the new book, the cautionary note is now on Page 585, where no one is likely to see it.

There is also a not-so-clearly labeled warning about the use of such simplistic or predictive methods present in both editions. But again, this is given a few pages later and is easy to miss.
To Conclude
A quick online search today will bring up dozens of Analytical Tools and Websites promoting this simplistic and dangerous formula as the "Benjamin Graham Formula" and even discouraging use of the methods Graham actually recommended.
Thus, what started out as a simple editing mistake seems to have snowballed into a gross distortion of fact; turning what was to be a shining example of what not to do into an oft recommended "Graham method" today.
A more comprehensive article about this formula is now available at Understanding The Benjamin Graham Formula Correctly.
Watch Video
Submitted by GrahamValue. Created on Monday 23rd September 2013. Updated on Wednesday 29th January 2025.

Comments
Actually it is not so much
Actually it is not so much about the formula being 'wrong'. There is nothing really wrong about it- it is to get an approximate value IF you can anticipate growth accurately. However the issue is that anticipating/ estimating growth CANNOT be done reliably and consistently and thus the formula is wrongly applied. Nevertheless it can be utilized under the right application. In Graham's example, he used this formula to work backwards, using the Xerox and GM example. At that time the market wrongly expected Xerox to grow at a rate of 32.4% annually (which was obviously overly optimistic and unrealistic), and GM to grow at only 2.8% annually (which was way too pessimistic). In other words, using the formula, we can identify overvalued and undervalued companies when the market wrongly anticipated growth at either extremities (either too high or too low).
Absolutely
Exactly, Daniel Lim!
This formula is only useful to study overvaluations and undervaluations, retrospectively.
It cannot be used to calculate intrinsic values, or predict future growth rates. But that's what it's used for the most today.
However, there seems to be a
However, there seems to be a mistake with the calculation for the projected growth rate of Xerox. He uses the formula ( P/E ratio - 8.5 ) / 2 which leads to the correct numbers for the other examples, but not for Xerox! With the given P/E ratio of 25.0 for Xerox, you get a projected growth rate of 8.3, not 32.4! So, either he miscalculated or the P/E ratio printed in the book is wrong.
1963 Stock Split
Good point, Heiko.
The original edition of the book has the same number — 32.4 — as well.
Xerox underwent a stock split in 1963. This is mentioned as footnote under table 11-5.
This could be the cause of the discrepancy.